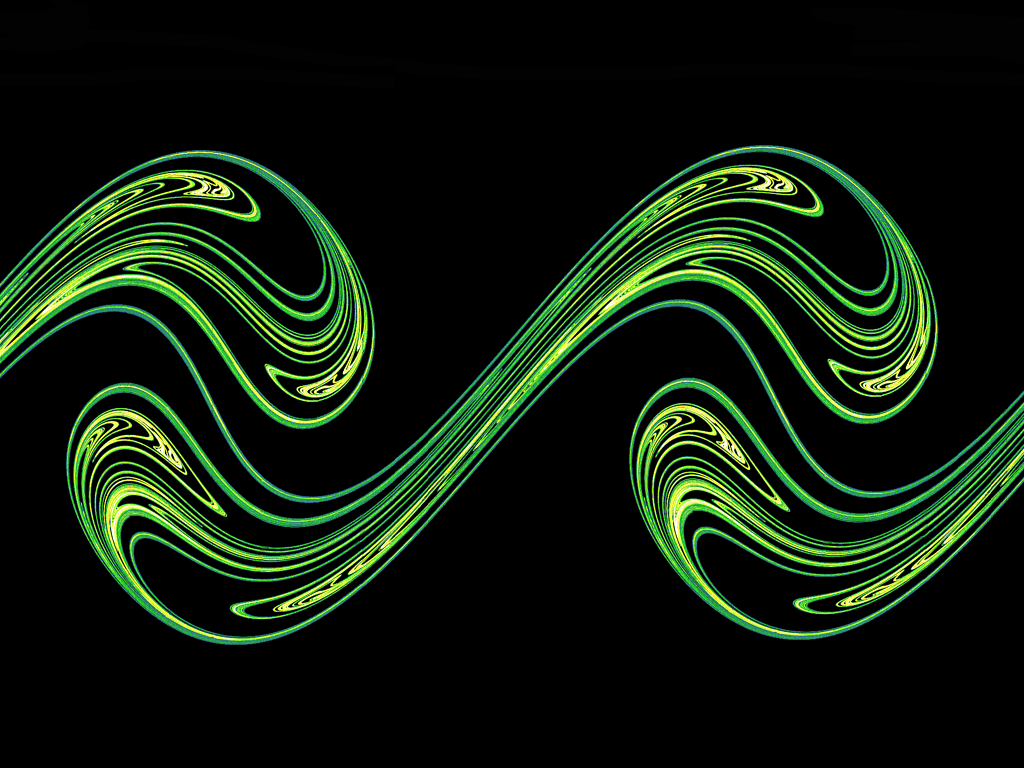
Feature 3, regular pattern in a specific view
This feature can be demonstared in precise experiments or numerical simulations only, run for a long time. It is worth the trouble to use a view of the motion which plots the velocity in a given moment as a function of the position. As time passes, the point representing these two data on a plane moves on, and a curve is traced out.
This curve is nothing but plotting the velocity as a function of the position (angle) at any instant in which our sensor records a value (with a time difference of 0.1 s). A complicated coil is drawn up, illustrating the irregular nature of chaos. Red dots indicate the instants when a full period of oscillation is over (when the suspension point is in its rightmost position). These points appear to show up randomly,
If, however, this sampling is applied for a long time, and only the red points are plotted, an intricate pattern evolves.
The initially seemingly random appearance of points starts, after some time, accumulate in certain regions, and avoiding certain others. It is remarkable to see that a kind of order appears in this view of chaos: a huge number of points are allowed to occur in this plane during the chaotic motion (but many others - the points of the white regions - are forbidden).
More details of the mentioned order in chaos is unfolded when following the motion of our ruler by means of a computer simulation (where the lack of sensor-related restrictions allow us to take the time difference much smaller than 0.1 s).
If interested in how the point representing the velocity-position pairs on a plane moves on in a simulation, click here.
You cen check the sequence of snapshots taken in the the simulation at the rightmost position of the suspension point to discover that the points trace out complicatedly interwoven filaments with empty regions between them, if you click here.
How this picture looks when obtained in a long numerical run, artistically colored, is worth checking by clicking here.
The pattern provides an example of what is called a chaotic attractor. The object is called an attractor since all motions are attracted to it. Due to friction, the system forgets its initial state, the same set of points emerges in this sampling procedure after some time, irrespective of where the motion was intitated. There is an 'infinite' number of permitted states at the sampled instances, extending over a large range. This is in harmony with the fact that the chaotic motion is aperiodic. It is surprising, however, that the plethora of the permitted states is ordered in some sense. Such complicated patterns are examples of fractals, an unusual class of geometric objects.
If we blow up a square from the pattern of the chaotic attractor and repeat this blowing-up once again
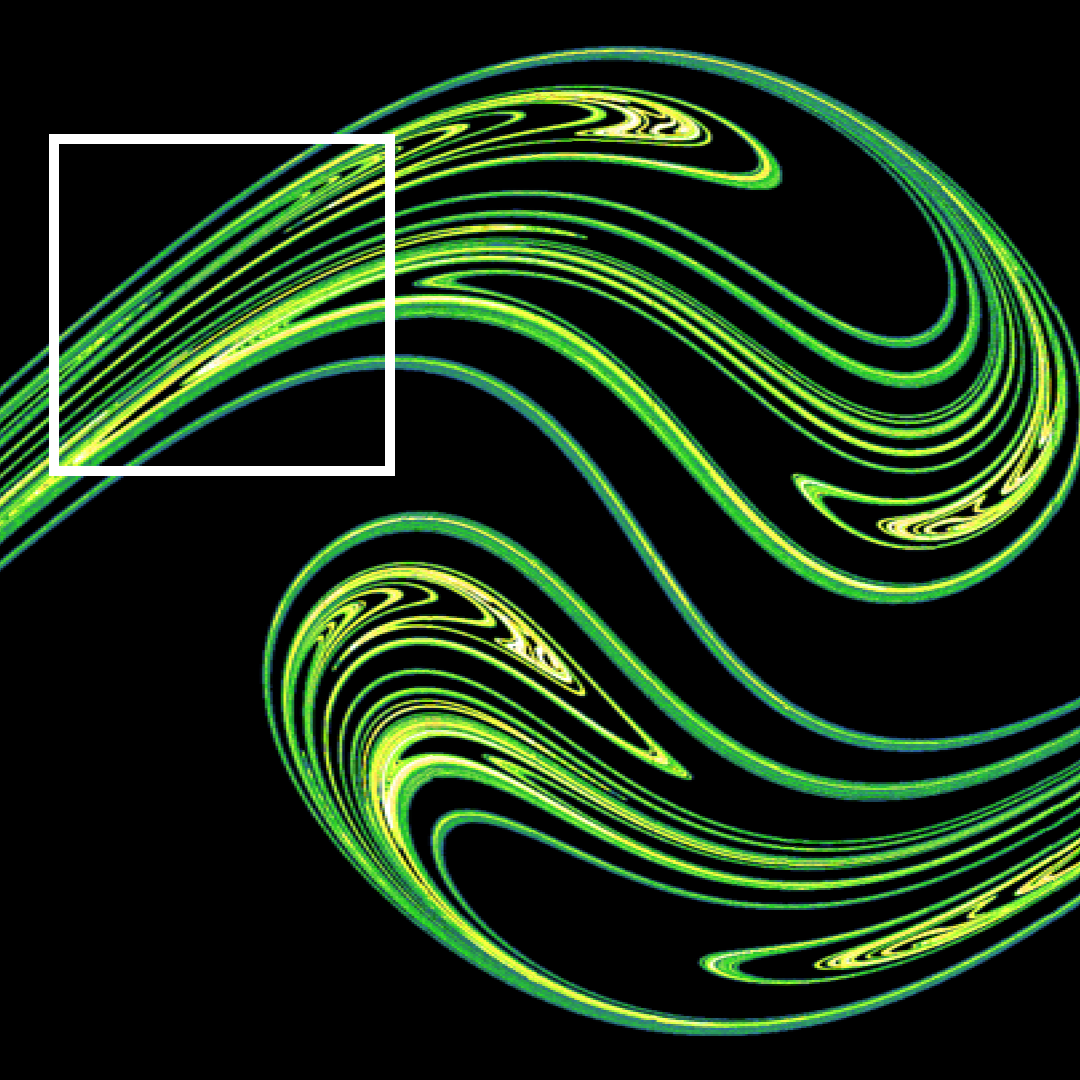

the latter ones appear to be nearly identical. We say, the geometry is self-similar. This self-similarity is the definitive property of fractal objects. This picture illustrates that the chaotic attractor is a bundle of approximately parallel line on smaller and smaller scales. Chaos is thus related to filamentary fractals.
The fractal pattern identified also indicates that chaos is not as random as noise. This is so since a motion is always a consequence of some laws, such as the Newtonian equations of motion in physics.
Based on the three features discussed, try to give an anser as detailed as possible to the question: what is chaos?
Chaos is a long lasting motion of relatively simple systems, which is
- irregular in time, truly aperiodic, and is not the superposition of periodic components
and
- sensitive to initial conditions: small initial differences are strongly magnified, and is unpredicable
and
- in an appropriate view not fully irregular, appears to be structured but complex, exhibits a fractal pattern.
Do the features explored up to now hold true for the periodic motions learnt in your physics curriculum?
No, they are predictable and are not related to fractals is any way.
We saw that a slight modification of a familiar case converts the motion into chaos. If you wish to have an overview of all possible motions of simple systems, you cannot avoid becoming acquainted with the unusual features of chaos.