Feature 4, probabilitic view, or How to cope with unpredictable systems?
It is certainly an unusual feature that the state of chaotic systems cannot be predicted for a time interval longer than the prediction time. What is then a proper long term scientific description of such systems? The answer follows from the 3 basic features discussed above. The existence of the chaotic attractor implies that only certain states, the fractal set of points of the attractor (to which all motions converge to), are permitted. We do not know which of the permitted states will belong to a given motion, but we can ask about the probability with respect to which a permitted state (e.g. the state with the fatest possible rotation of the ruler) occurs among all possible motions.
The analysis of chaotic systems teaches us that from a statistical point of view the motion developing on the attractor can be described with full accuracy. Even if individual motions are unpredictable, the statistical properties of all possible motions can be predicted! The somewhat pessimistic view of interpreting the 'butterfly effect' as not being able to be sure of anything, should be counterbalanced by the potentially perfect ability for a statistical characterization of chaos. (We are then not surprised by seeing weather [or most recently even climatic!] forecasts moving in the direction of providing likelyhoods rather then precise predicted data - this is the proper treatment of such problems.)
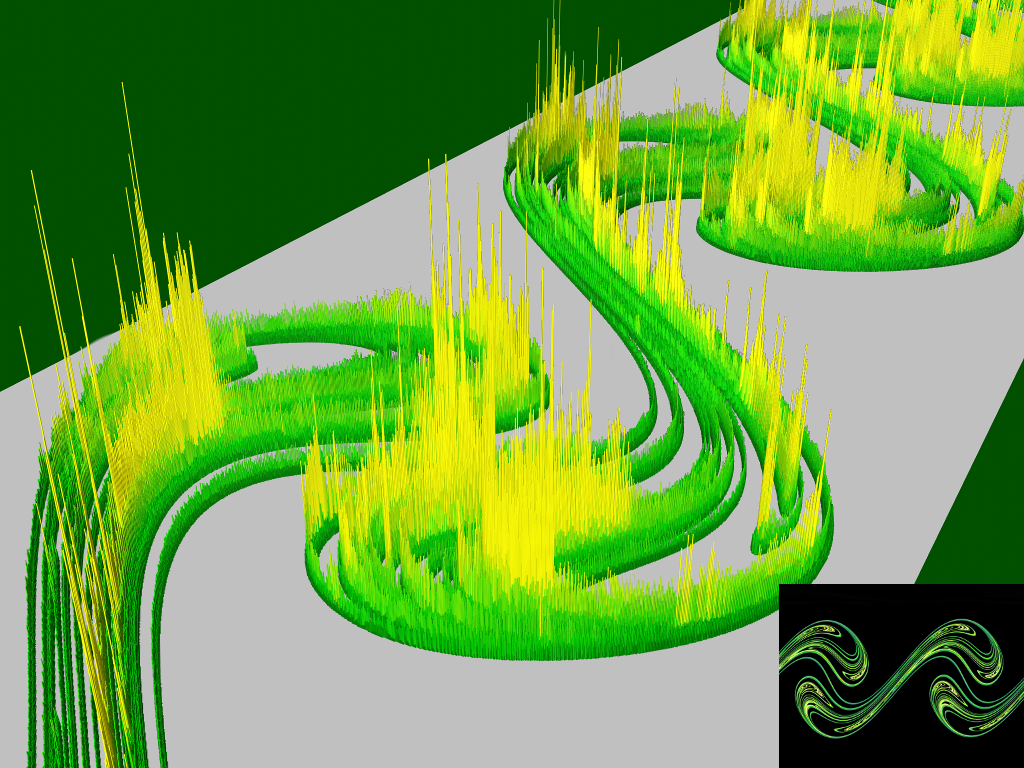
If interested in the probability with which different regions on the attractor of the chaotic pendulum is visited, click here, where, for a more artistic view, the angle is not restricted to -180, +180 degress, it grows continuously.
The distributon is rather uneven: the probability of a given state might be completely different from that of the neighboring pixel. This fine dependence on the states is an additional property of chaos. Note that such distributions are the consequences of the underlying equations. In our pendulum example from mechanics, the existence of the probability distribution follows from the Newtonian equation of motion!
What is a probability distribution like when the system is periodic and is observed at integer multiples of the period?
A single peak belonging to the point representing the location and velocity of the motion in the sampling procedure chosen.
Based on all four features discussed, try to give an anser as detailed as possible to the question: what is chaos?
Chaos is a long lasting motion of relatively simple systems, which is
- irregular in time, truly aperiodic, and is not the superposition of periodic components
and
- sensitive to initial conditions: small initial differences are strongly magnified, and is unpredicable
and
- in an appropriate view not fully irregular, appears to be structured but complex, exhibits a fractal pattern.
and
- its long-time behavior can fully be predicted by giving the probabiity distribution of the permitted states.
As the conjunctive and indicates, the listed characteristics are present simultaneously: when a simple system is aperiodic over a long time, its evolution must be unpredictable, can be represented by a fractal structure in suitable coordinates, but the long time motion can be properly characterized by means of probabilities.